
Middle row: small coupling: cold → hot temperature ( μ = 8 × 10 − 9 n ¯ = 0 → n ¯ = 10 15 the colors in the last panel are scaled by 1 0 8 in accordance with the axis scaling). Top row: Cold temperature: small → large coupling ( n ¯ = 0 μ = 6 → μ = 8 × 10 − 9). The blue dotted line indicates ℓ 1 sensitivity of a coherent state at zero temperature.
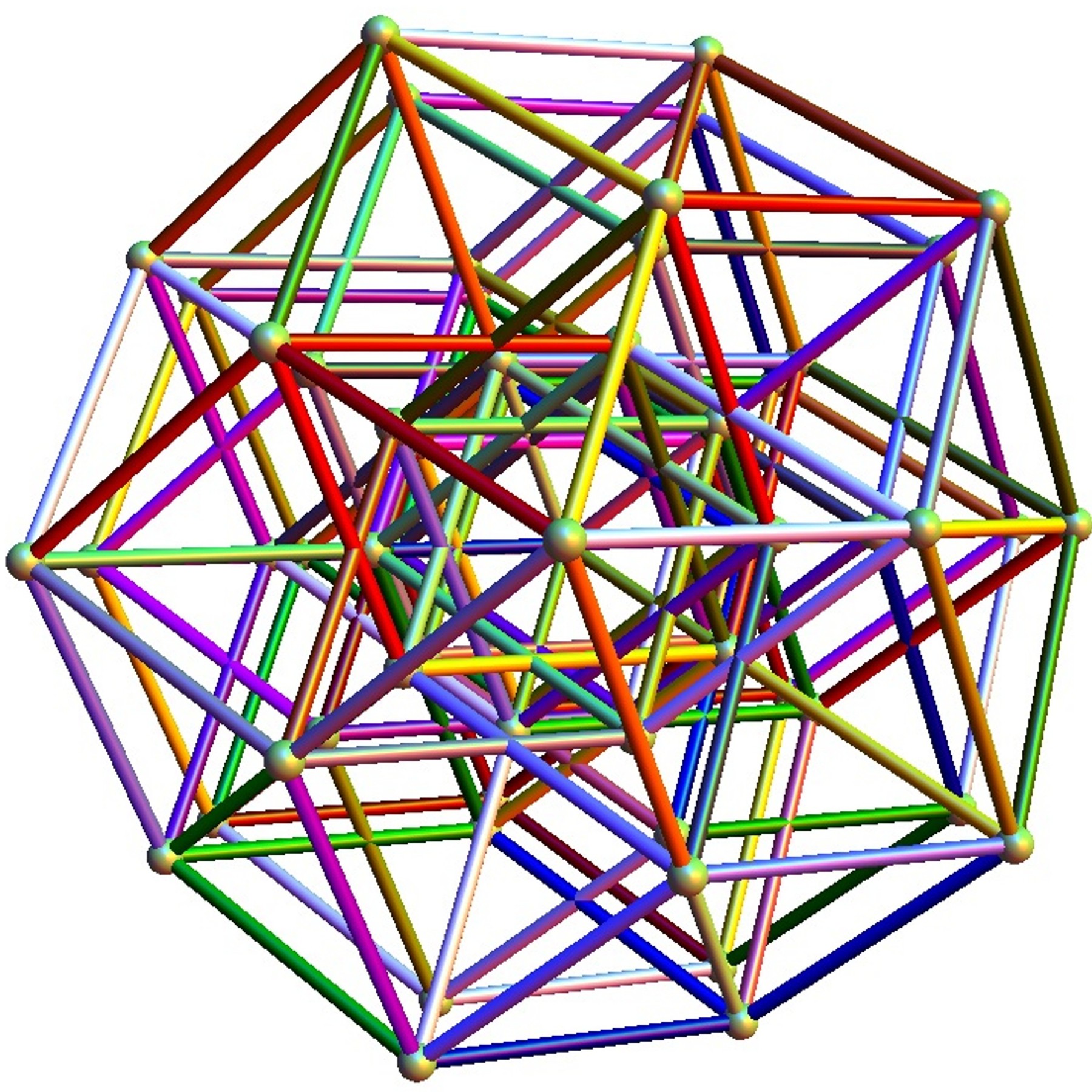
Right-hand side: Corresponding ℓ 1 sensitivity, d ℓ 1 / d σ, vs position or momentum displacement plots (position, pink horizontal triangles) or (momentum, black vertical triangles). All axes are in terms of the ground state width. The color bar on the bottom shows the value of the Wigner function where blue and green indicate areas of negative quasiprobability. Left-hand side: Theoretical density plots of the Wigner function in position-momentum ( X − P) phase space. 165–172.N = 2 hypercube state for a mechanical resonator as produced by our scheme. Evans, Pancyclicity of Möbius cubes with faulty nodes, Microprocessors and Microsystems, 30 ( 2006), pp. IJCMAT 0020-7160 Crossref ISI Google Scholar IFPLAT 0020-0190 Crossref ISI Google Scholar Xu, Fault-tolerant pancyclicity of augmented cubes, Inform. TCSCDI 0304-3975 Crossref ISI Google Scholar Tsai, Linear array and ring embeddings in conditional faulty hypercubes, Theoret. Kim, Panconnectivity and pancyclicity of hypercube-like interconnection networks with faulty elements, Theoret. Chwa, Recursive circulants and their embeddings among hypercubes, Theoret. Park, Panconnectivity and edge-pancyclicity of faulty recursive circulant $G(2^,4)$, Theoret. Park, Unpaired many-to-many disjoint path covers in hypercube-like interconnection networks, J. Xu, Panconnectivity and edge-fault-tolerant pancyclicity of augmented cubes, Parallel Comput., 33 ( 2007), pp. Fu, Fault-free Hamiltonian cycles in crossed cubes with conditional link faults, Inform. JOSUED 0920-8542 Crossref ISI Google Scholar Wu, Optimal fault-tolerant Hamiltonicity of star graphs with conditional edge faults, J. ITDSEO 1045-9219 Crossref ISI Google Scholar Lee, Conditional edge-fault Hamiltonicity of matching composition networks, IEEE Trans. Chen, Pancyclicity in Möbius cubes with maximal edge faults, Parallel Comput., 30 ( 2004), pp. ITCOB4 0018-9340 Crossref ISI Google Scholar Chang, Hamiltonian path embedding and pancyclicity on the Möbius cube with faulty nodes and faulty edges, IEEE Trans. Chen, Embedding fault-free cycles in crossed cubes with conditional link faults, J.
Fu, Fault-free Hamiltonian cycles in twisted cubes with conditional link faults, Theoret. Efe, The crossed cube architecture for parallel computation, IEEE Trans. Chedid, On the generalized twisted cube, Inform. Lee, On the existence of Hamiltonian circuits in faulty hypercubes, SIAM J. JGTHDO 0364-9024 Crossref ISI Google Scholar Tindell, Circulants and their connectivities, J. Stewart, Fault-tolerant embeddings of Hamiltonian circuits in k-ary n-cubes, SIAM J.


 0 kommentar(er)
0 kommentar(er)
